Temperature changes during a measurement with strain gauges can frequently have undesirable effects on the measurement result. Fortunately a number of options are available – with the "right" choice of a strain gauge, the one that suits the application best, by making use of the effects of a Wheatstone bridge circuit with a half or full bridge circuit, and also with computational methods – to compensate largely for unwanted temperature effects.
Please be aware: The temperature range of foil strain gauges is limited by the materials that are used. The maximum range is about 300°C to 400°C. If measurements have to be conducted at higher temperatures, high-temperature strain gauges that work according to different principles must be used. Temperature limits of HBM strain gauges are:
Of course the temperature limit of the adhesive you are using must also be observed. If an adhesive becomes soft as the temperature rises, the strain will no longer be accurately transfered. Temperature limits of HBM strain gauge adhesives are:
|
Influence quantity |
Possible compensating measure |
| Material expansion | Use self-compensated strain gauges |
| Cable resistance | Use multiwire techniques |
| Temperature coefficient of the gauge factor | Very low, usually ignored. Computational compensation while simultaneously measuring the temperature is possible. |
| Temperature influence on the modulus of elasticity | Usually ignored |
| The following points, which may also be related to temperature, are also relevant: | |
| Self-heating of the strain gauge | Observe the maximum excitation voltage |
| Climate/relative humidity | Careful covering of the measuring point |
| Adhesive creep | Observe the temperature limits of the adhesive you are using |
Special attention should be paid to these two influence quantities:
In addition to these two main factors, there are other influence quantities for which temperature effects play a role. The sum of these effects can be ignored, however, and can usually be resolved through computational compensation (see the explanation of computational compensation below).
Materials that are being measured expand as the temperature increases. This expansion is described by the expansion coefficient of the material. The value depends on the material. For steel it is approximately 11 ppm/K, for example, meaning an expansion of 11 µm/m for a thermal difference of plus/minus one degree Celsius. Material expansion, influenced by changes in temperature, ultimately results in measuring an "apparent" strain, in other words a strain with no load.
Change in volume
Learn how the coefficient of thermal coefficient of expansion of aluminium can be determined using "mismatched" foil strain gauges.
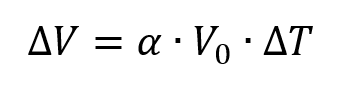
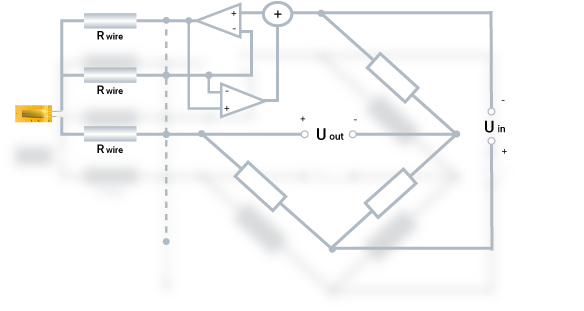
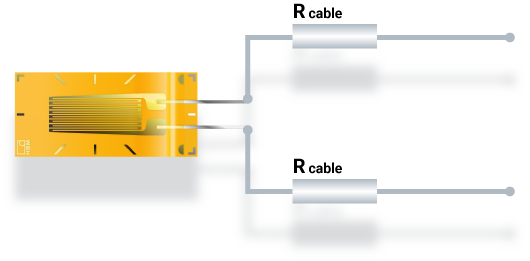When a two-conductor circuit is used (see diagram) the resistance of a measurement cable is added to the resistance of the strain gauge – and therefore influences the measurement. In addition to the resulting zero drift and the reduction of the effective gauge factor, the resistance of the measuring cable is also temperature-dependent.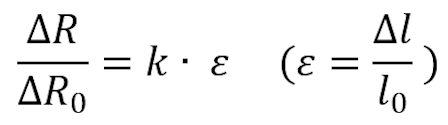
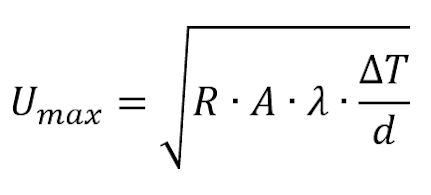
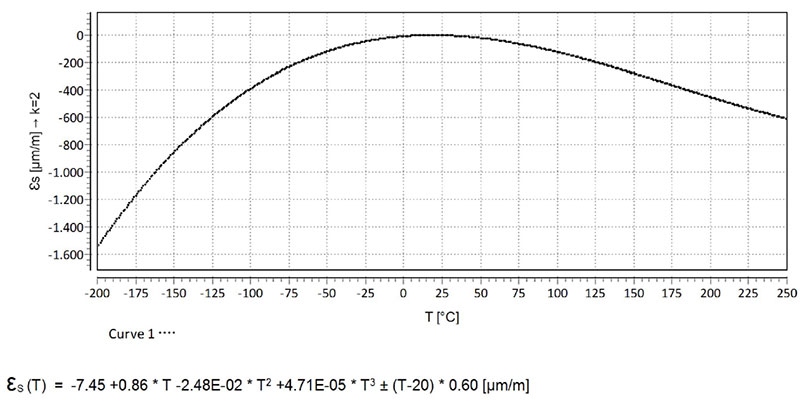
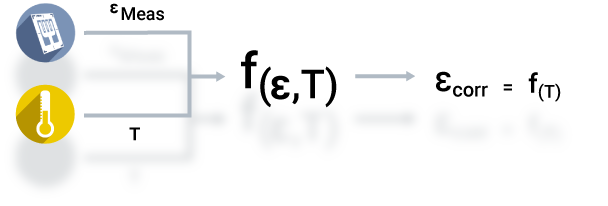
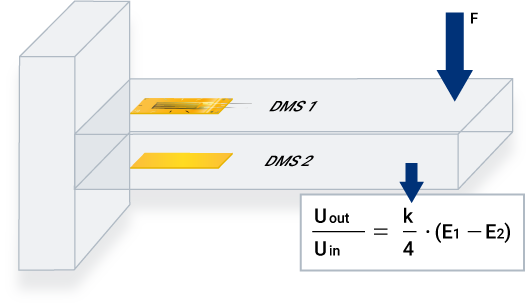A suitable counter measure in this case is the use of multiwire techniques as described below.
The gauge factor is the most important property of the strain gauge. It describes the correlation between strain and change in resistance. The gauge factor is temperature-dependent. With typical temperature coefficients of the gauge factor of 0.01 %/K, its distorting effect on the measurement result is usually relatively small and is therefore mostly ignored. However, a computational compensation (for the temperature measurement) is also feasible.
The modulus of elasticity is a material-dependent property of the measuring body. It describes the correlation between the measured strain and the mechanical stress. The modulus of elasticity is temperature-dependent. A typical value for steel in this case is approx. -0.02%/K. In experimental stress analysis, the effect of the modulus of elasticity is typically ignored. With high-precision transducers that can be calibrated, compensation is made by means of temperature-dependent nickel elements in the bridge.
The excitation voltage causes the strain gauge to heat up in comparison to the measuring body. Depending on the thermal conductivity of the measuring body, heat conductance is more or less absorbed by the measuring body. If the measuring body conducts heat poorly, the result may be a difference in temperature between the measuring body and the strain gauge. This could possibly interfere with the functioning of the self-compensated strain gauge.
If the measuring point is not adequately protected, a drift in the zero point may occur depending on the relative humidity. This is due to water molecules of the adhesive and strain gauge carrier material being absorbed (hygroscopy). A suitable counter measure is carefully covering the measuring point.
As the temperature increases, adhesives become soft and are no longer able to transfer 100% of the strain. In this way they are comparable to a declining gauge factor. Because of this it is important to always observe the temperature limits of the adhesive and to choose them appropriately for the field of application.
Self-compensated strain gauges are specially developed to compensate for the temperature behavior of certain materials by their own temperature behavior. This means that they counteract the apparent strain (and thus the temperature-induced expansion of the measuring body). Therefore a strain gauge with a temperature response suitable for the material of the measuring body is selected.
Temperature adjustments for commonly used materials with self-compensated strain gauges:
Selecting a strain gauge that is adapted to the material compensates for the lion's share of apparent strain. A residual error remains (non-linear component). This error is determined during production and is included in the data sheet (see illustration). For more extensive calculations, for example with greater temperature changes, you can also perform a computational compensation (see below).
LUnderstand the ¼-bridge compensation calculation step by step based on a practical example.
Along with the use of self-compensated strain gauges, connecting to a half or full bridge circuit as well as the use of a three or four-wire circuit is another important method of compensation which is especially useful for minimizing or even completely eliminating the effect of cable resistance.
The Wheatstone bridge circuit converts very small changes in resistance into a measurable electric voltage. The four resistors can be replaced by one (quarter bridge circuit), two (half bridge circuit) or four (full bridge circuit) strain gauges.
Since the individual branches flow with different signs in the Wheatstone bridge circuit, there is a possibility for compensation. This temperature compensation effect can be demonstrated based on the example of a bending beam:
Under positive load, the spring exhibits strain (+) on the top and compression (-) on the bottom. If two strain gauges are connected to a Wheatstone bridge circuit, the result is to double the signal. If temperature-dependent strain occurs, the strain appears to both strain gauges with the same sign. Thus the effects cancel each other out in the Wheatstone bridge circuit.
The effect of cable resistance can be largely compensated for selectively by a three wire circuit. To do this the supply lead and an additional third lead are wired into different branches of the Wheatstone bridge circuit. Since the two cables behave oppositely due to the symmetry of the structure and thus compensate for each other mutually, the cable resistances are compensated for by the three wire circuit, except in the case of asymmetrical cables and with temperature gradients.
All cable effects are even compensated for by the HBM's patented four-wire circuit.
Computational compensation can be performed for the residual error with self-compensated strain gauges, for the error of a strain gauge that is not adjusted or is poorly adjusted, and also for other small errors (such as the temperature dependence of the gauge factor).
To do this the temperature is measured in parallel and the measured strain is corrected by a corresponding online or subsequently calculated channel. The temperature gradients must also be considered. Multiple measuring points must be provided for the temperature if necessary. Software tools such as catman® from HBM also offer appropriate functions for computational compensation.
In addition to the sensor itself, the amplifier also plays an important role in considering temperature influences. This applies especially with thermoelectric voltages: Due to the thermoelectric effect, a temperature-dependent electric voltage is produced where different materials are connected. Thermocouples make use of this effect. However, this also has an effect on a strain gauge measurement system (temperature-dependent zero error (zero signal return)).
Thermoelectric voltage can be largely compensated for by using a carrier-frequency amplifier such as QuantumX MX1615B or QuantumX MX1616B from HBM. In this case there is a sinusoidal excitation voltage so that the measurement signal can be modulated to a periodic signal. Demodulation is performed digitally after the signal passes a band pass filter so that the quasi-static thermoelectric voltages can be filtered out on the way to the amplifier.
Depending on the influence quantity, various options are available for minimizing the influence of temperature effects on the measurement result. Here is your checklist for measurements with low temperature influence:
This will bring together HBM, Brüel & Kjær, nCode, ReliaSoft, and Discom brands, helping you innovate faster for a cleaner, healthier, and more productive world.
This will bring together HBM, Brüel & Kjær, nCode, ReliaSoft, and Discom brands, helping you innovate faster for a cleaner, healthier, and more productive world.
This will bring together HBM, Brüel & Kjær, nCode, ReliaSoft, and Discom brands, helping you innovate faster for a cleaner, healthier, and more productive world.
This will bring together HBM, Brüel & Kjær, nCode, ReliaSoft, and Discom brands, helping you innovate faster for a cleaner, healthier, and more productive world.
This will bring together HBM, Brüel & Kjær, nCode, ReliaSoft, and Discom brands, helping you innovate faster for a cleaner, healthier, and more productive world.